
CBSE Class 9 Maths Notes Chapter 11: CBSE Class 9 Maths Notes Chapter 11 describes how to draw various forms with a ruler and compass. This chapter explains how to construct the bisector of a given angle and how to construct a perpendicular bisector of a given line segment.
Construction instructions and a clear illustration are provided. To get good grades, you should also complete all of the class 9 Maths Chapter 11 building problems in the textbook.The important topics and subtopics covered under Constructions class 9 are:
CBSE Class 9 Maths Notes Chapter 11 PDF
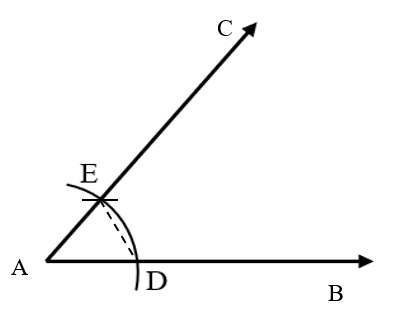
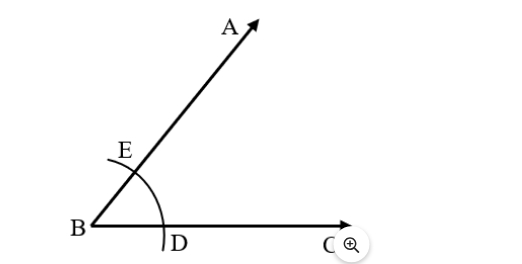 Create arcs with E and D as their centers and a radius greater than 1 by 2 DE so that they cross at F.
Create a ray that now connects B and F and extends it. Therefore, the necessary angle bisector is BF.
Create arcs with E and D as their centers and a radius greater than 1 by 2 DE so that they cross at F.
Create a ray that now connects B and F and extends it. Therefore, the necessary angle bisector is BF.
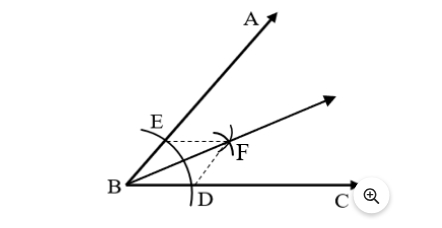

 To create a 60∘ angle at a given ray's starting point, take a ray AB with an initial point of A. From there, draw another ray AC so that ∠BAC=60∘. The actions that need to be taken are as follows:
Using A as the center, sketch an arc that intersects the ray AB at point D. Now, draw two arcs that intersect at a point, let's say E, using A and D as the centers and AD as the radius.
Connect AE and extend it to point C on the AC ray. Therefore, CAB=60∘.
To create a 60∘ angle at a given ray's starting point, take a ray AB with an initial point of A. From there, draw another ray AC so that ∠BAC=60∘. The actions that need to be taken are as follows:
Using A as the center, sketch an arc that intersects the ray AB at point D. Now, draw two arcs that intersect at a point, let's say E, using A and D as the centers and AD as the radius.
Connect AE and extend it to point C on the AC ray. Therefore, CAB=60∘.
Some Construction of Triangles:
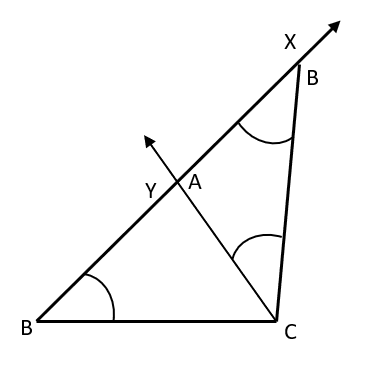
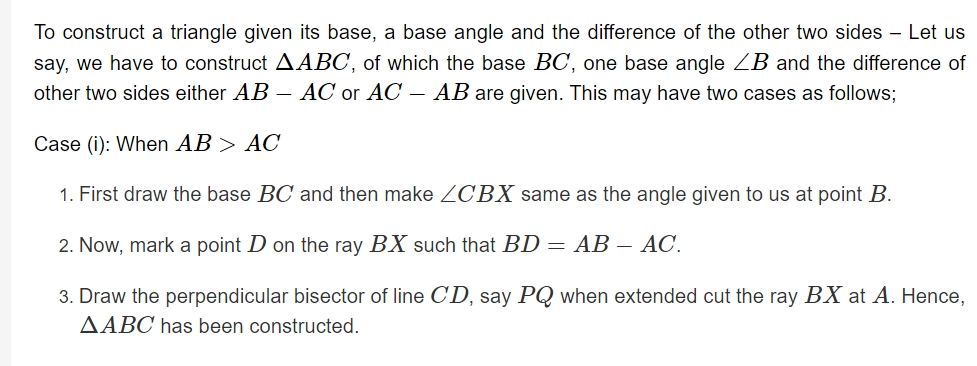
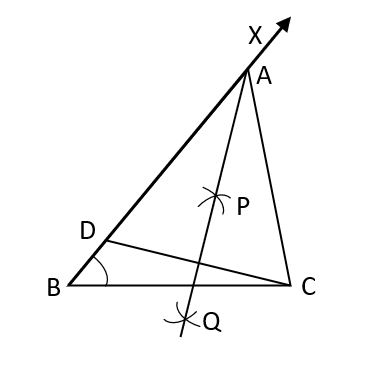
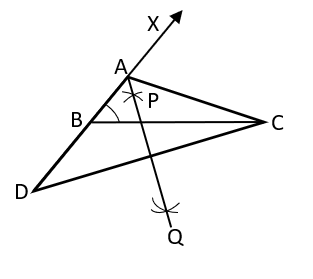
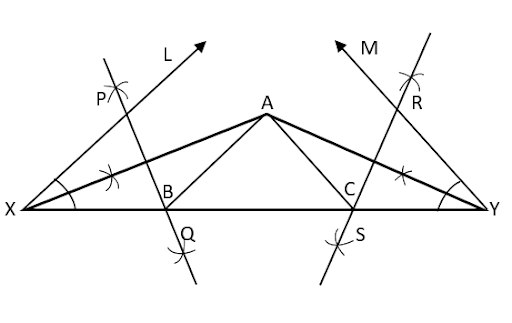
To create a triangle, measurements of at least three of its components are needed. However, none of the three-element combinations is sufficient for the goal. For instance, given the dimensions of the aspects and an uncovered perspective among the offered aspects, it is not possible to create a unique triangle. When (i) the base, one base attitude, and the total of the opposing aspects are provided, a triangle can be constructed. (ii) the basis, a foundational mindset, and the differentiation between the opposing elements are provided (iii) The base and perimeter angles are provided.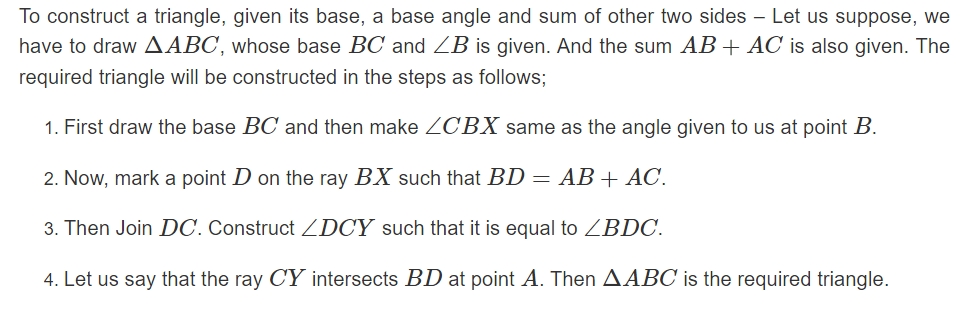

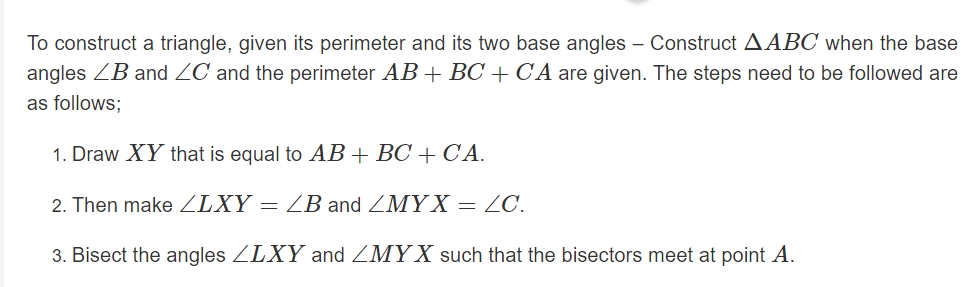


Concept Clarity: CBSE Class 9 Maths Notes Chapter 11 helps students to understand the chapter better. Students can gain a firm grasp of these ideas.
Problem-solving abilities: The notes provide students with a range of practice problems and examples to aid in the development of their problem-solving abilities. Students can strengthen their grasp of ideas and develop their problem-solving skills by completing these notes.
Structured Learning: The notes are arranged methodically, covering subjects one after the other. This facilitates pupils' ability to learn logically.
Exam Preparation: CBSE Class 9 Maths Notes Chapter 11 is tailored to match the format of the exams. Students can improve their performance in the mathematics portion and successfully prepare for their exams by going over these notes.
