CIRCUMFERENCE OF A CIRCLE

CIRCLE:

The collection of all the points in a plane, which are at a fixed distance from a fixed point in the plane, is called a circle.

The fixed point is called the centre of the circle and the fixed distance is called the radius of the circle.

A circle is a geometric figure in a plane such that all its points are equidistant from a fixed point in a plane. The fixed point is the centre of the circle and the constant distance from the centre is the radius of the circle.

A circle has center O and radius r is usually denoted by C (O, r). Therefore in set notation, we write
C(O, r) = {X; OX = r}.

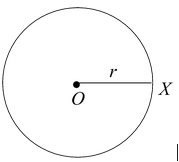
In figure, O is the centre and the length OX is the radius of the circle. So the line segment joining the centre and any point on the circle is called a radius of the circle.

CIRCUMFERENCE OF A CIRCLE:

The circumference of a circle is the length of the complete circular curve constituting the circle.

CHORD OF A CIRCLE:

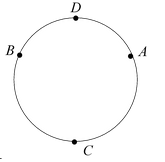
ARC OF A CIRCLE:

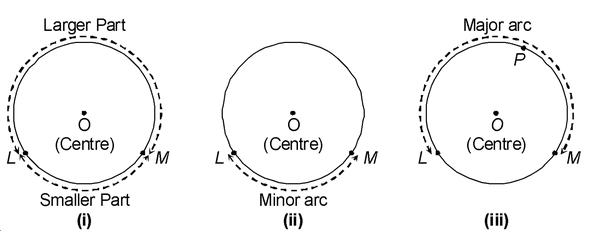
Any two points A and B of a circle, divide the circle in two parts as shown in figure (i). The smaller part is called a minor arc of the circle as shown in figure (ii), and it is denoted by . The larger part is called a major arc as shown in figure (iii). It is denoted by where P is a point on this part of the arc. 

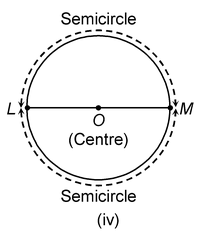
In case the two parts are equal, then we find LM is a diameter of the circle and two parts of the circle are equal. Each part of the circle as shown in figure (iv) is a semicircle.

Length of an arc

The length of an arc 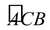is the length of the fine thread which just covers the arc completely. If 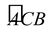is the major arc and is the minor arc, then we get that

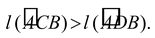
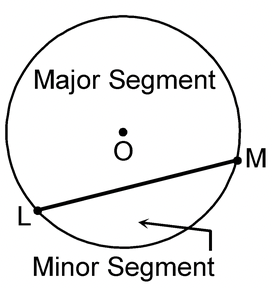
A circle divides a plane into three parts, interior, exterior and the circle itself. The interior region is called the circular region. A chord divides a circular region into two parts, called the segments of the circular region or the segments of the circle. The smaller segment is called the minor segment and the large one is called the major segment. In case the two segments are equal, then each is called a semicircular region.

 

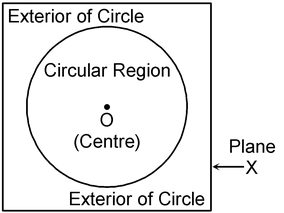
INTERIOR OF A CIRCLE:

The plane region lying in the circle as shown in figure is called the interior of the circle.

The circle and its interior both as a whole constitutes the circular region.

SEGMENT OF A CIRCULAR REGION:

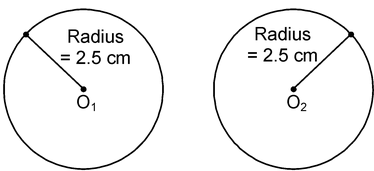
CONGRUENT CIRCLES:

Two circles are congruent to each other if and only if they have equal radii.

In the figure two circles have equal radii. Therefore, the two circles are congruent to each other.

DIAMETER(d):

The chord which passes through the centre of the circle, is called a diameter of the circle.

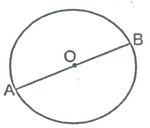
A diameter is the longest chord and all diameter have the same length, which is equal to two times the radius. In figure, AOB is a diameter of circle.

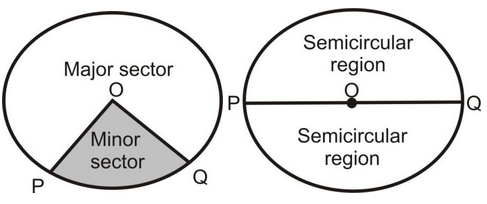
SECTOR (h): 

The region between an arc and the two radii, joining the centre to the end points of the arc is called a sector. Like segments, we find that the minor arc corresponds to the minor sector and the major arc corresponds to the major sector. In figure, the region OPQ in the minor sector and the remaining part of the circular region is the major sector. When two arcs are equal, then both segments and both sectors become the same and each is known as a semicircular region.

THEOREM1:

Equal chords of a circle subtend equal angles at the centre.

Given :AB and CD are the two equal chords of a circle with centre O.

To Prove :∠AOB = ∠COD.

Proof :In ΔAOB and ΔCOD,

OA = OC [Radii of a circle] 

OB = OD [Radii of a circle]

AB = CD [Given]

ΔAOB ≅ΔCOD [By SSS]

∠AO B = ∠COD. [By cpctc]

Converse of above Theorem:

In the angles subtended by the chords of a circle at the centre are equal, then the chords are equal.

Given :∠AOB and ∠POQ are two equal angles subtended by chords AB and PQ of a circle at its centre O.

To Prove :AB = PQ

Proof :In ΔAOB and ΔPOQ,

OA = OP [Radii of a circle]

OB = OQ [Radii of a circle]

∠AOB = ∠POQ [Given]

ΔAOB≅ΔPOQ [By SAS]

AB = PQ [By cpctc] Hence Proved.

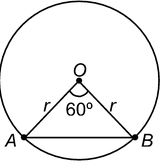
question 1 AB is a chord of a circle having centre at O. If ∠AOB = 60º, prove that the length of chord AB is equal to radius.

From (i) and (ii), ∠A = ∠B = 60º

Thus,∠O = ∠A = ∠B = 60º

⇒ ΔOAB is an equilateral triangle.

⇒ AB = OA = OB = r.

i.e., AB = r

THEOREM2:

The perpendicular from the centre of a circle to a chord bisects the chord.

Given:  A circle with centre O. AB is a chord of this circle. OM AB.

To Prove :MA = MB.

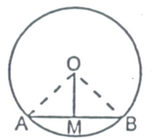
Construction :Join OA and OB.

Proof :In right triangles OMA and OMB,

OA = OB [Radii of a circle]

OM = OM [Common]

∠OMA = ∠OMB [90o each]

ΔOMA≅ΔOMB [By RHS]

MA = MB [By cpctc] Hence Proved.

Converse of above Theorem:

The line drawn through the centre of a circle to bisect a chord a perpendicular to the chord.

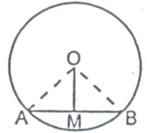
Given :A circle with centre O. AB is a chord of this circle whose mid-point is M.

To Prove :OM AB.

Construction :Join OA and OB.

Proof :In ΔOMA and ΔOMB. 

MA = MB [Given]

OM = OM [Common]

OA = OB [Radii of a circle]

ΔOMA≅ΔOMB [By SSS]

∠AMO = ∠BMO [By cpctc]

But ∠AMO + ∠BMO = 180o [Linear pair axiom]

∠AMO = ∠BMO = 90o

⇒ OM AB.

THEOREM 3:

There is one and only one circle passing through three given non-collinear points.

Proof: Let us take three points A, B and C, which are not on the same line, or in other words, they are not collinear [as in figure]. Draw perpendicular bisectors of AB and BC say, PQ and RS respectively. Let these perpendicular bistros intersect at one point O. (Note that PQ and RS will intersect because they are not parallel) [as in figure]. 

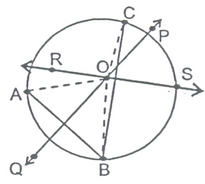
O lies on the perpendicular bisector PQ of AB.

OA = OB

[Every point on the perpendicular bisector of a line segment is equidistant from its end points]

Similarly,

O lies on the perpendicular bisector RS of BC.

OB = OC

[Every point on the perpendicular bisector of a line segment is equidistant from its end points]

So, OA = OB = OC

i.e., the points A, B and C are at equal distances from the point O.

So, if we draw a circle with centre O and radius OA it will also pass through B and C. This shows that there is a circle passing through the three points A, B and C. We know that two lines (perpendicular bisectors) can intersect at only one point, so we can draw only one circle with radius OA. In other words, there is a unique circle passing through A, B and C.

Hence Proved.

Things to remember :

If ABC is a triangle, then by above theorem, there is a unique circle passing through the three vertices A, B and C of the triangle. This circle the circumcircle of the ΔABC. Its centre and radius are called respectively the circumcentre and the circumradius of the triangle.

question 2 In the given figure, AB is the diameter of the circle. If C is a point on the circle such that ADC = 700, then BAC is

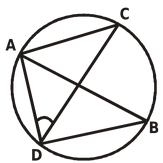
(a) 200 (b) 600 (c) 700 (d) 300

Solution: Given AB is the diameter, ADC = 700

As AB is the diameter of the circle, ADB = 900 ( Angle in a semicircle = 900)

BDC = ADB – ADC = 900 – 700 = 200

andBAC = BDC ( As angles in the same segment are equal)

BAC = 200

question 3 In figure, AB = CB and O is the centre of the circle. Prove that BO bisects ABC.

Solution: Given: In figure, AB = CB and O is the centre of the circle.

To Prove: BO bisects ABC.

Construction: Join OA and OC.

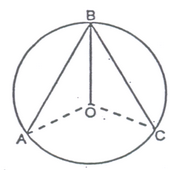
Proof :In ΔOAB and ΔOCB,

OA = OC [Radii of the same circle]

AB = CB [Given]

OB = OB [Common]

ΔOAB≅ΔOCB [By SSS]

∠ABO = ∠CBO [By cpctc]

⇒ BO bisects ∠ABC. Hence Proved.

question 4 A, B and C are three points of a circle whose centre is not indicated. How will you located the centre.

Solution: A, B and C are three points. Join AB, BC and AC. Draw their perpendicular bisectors, these perpendicular bisectors will pass through centre will meet at O which will be centre.

 

question 5 Two circles with centres A and B intersect at C and D. Prove that ∠ACB = ∠ADB.

Solution: Given :Two circles with centres A and B intersect at C and D.

To Prove :∠ACB = ∠ADB.

Construction :Join AC, AD, BC, BD and AB.

Proof :In ΔACB an ΔADB,

AC = AD [Radii of the same circle]

BC = BD [Radii of the same circle]

AB = AB [Common]

ΔACB≅ΔADB [By SSS]

∠ACB = ∠ADB. [By cpctc] Hence Proved.

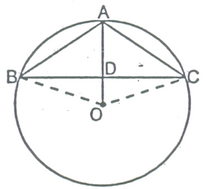
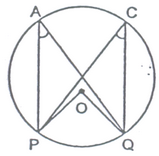
question 6 In figure, AB≅ACand O is the centre of the circle. Prove that OA is the perpendicular bisector of BC.

Solution: Given :In figure, AB≅AC and O is the centre of the circle.

To Prove :OA is the perpendicular bisector of BC.

Construction :Join OB and OC. 

Proof :

AB≅AC [Given]

chord AB = chord AC.

[If two arcs of a circle are congruent, then their corresponding chords are equal.]

∠AOB = ∠AOC ....(i)

[Equal chords of a circle subtend equal angles at the centre]

In ΔOBC and ΔOCD,

∠DOB = ∠DOC [From (1)]

OB = OC [Radii of the same circle]

OD = OD [Common]

ΔOBD≅ΔOCD [By SAS]

∠ODB = ∠ODC ....(ii) [By cpctc]

AndBD = CD ...(ii) [By cpctc]

But ∠BDC = 180^o

∠ODB + ∠ODC = 180^o

⇒ ∠ODB + ∠ODB = 180^o [From equation (ii)]

⇒ 2∠ODB = 180^o

⇒ ∠ODB = 90^o

∠ODB = ∠ODC = 90^o ....(iv) [From (ii)]

So, by (iii) and (iv), OA is the perpendicular bisector of BC. Hence Proved.

question 7 Two circles with centres A and B intersect each other at P and Q and M is the mid-point of PQ. Give reasons fro the following statements:

(i) AM PQ

(ii) BM 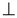PQ

(iii) A, M, B are collinear

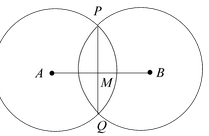
Solution: (i) M is the mid point of chord PQ. A is centre

 

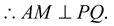
(ii) similar to (i)

(iii) A, M, B are collinear 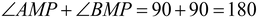by part (i) and (ii)

AMB is a straight line.

question 8 In the following figure, the bisectors of angles BAD and BCD intersect the circle at E and F respectively. If EFA = 400, then AEF is 

(a) 600 (b) 500 (c) 400 (d) 300

Solution: In the figure, if DAE = x and BCF = y.

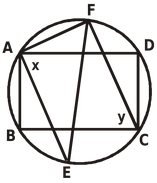
BAE = x0 and BCD = y0 ( A and C are the bisectors)

2DAE + 2DCF = 1800

⇒DAE + DCF =

i.e., x + y = 900

As angles in the segment are equal FAD = DCF = y

Hence EAF = EAD + DAF = 900

AEF = 1800 – (EAF + EFA) = 1800 – (900 + 400) = 500

THEOREM 4:

Equal chords of a circle (or of congruent circles) are equidistant from the centre (or centres).

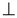Given: A circle have two equal chords AB & CD. .e. AB = CD and OM AB, ON CD

To Prove: OM = ON

Construction: Join OB & OD

Proof: AB = CD (Given)

[The perpendicular drawn from the centre of a circle to bisect the chord.]

 

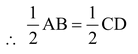
⇒ BM = DN

In ΔOMB &ΔOND

∠OMB = ∠OND = 90^o [Given]

OB = OD [Radii of same circle]

Side BM = Side DN [Proved above]

ΔOMB≅ΔOND [By R.H.S.]

OM = ON [By cpctc] Hence Proved.

Things to remember :

Chords equidistant from the centre of a circle are equal in length.

question 9 In a circle of radius 13 cm find the distance of a chord of length 24 cm, from the centre. 

If OM || BC, find BC.

Solution: Let PQ be a chord of a circle with centre O such that OP = 13 cm, PQ = 24 cm.

Let,OM PQ then PM = MB = 12 cm.

From right triangle OPM

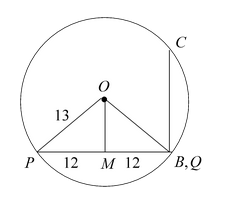
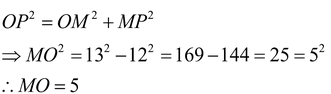
Distance of chord = 5 cm

Since

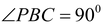
PC is a diameter

BC = 10 cm

question 10 AB and CD are equal chords of a circle whose centre is O. When produced, these chords meet at E. Prove that EB = ED.

Solution: Given :AB and CD are equal chords of a circle whose centre is O. When produced, these chords meet at E.

To Prove :EB = ED.

Construction :From O draw OP 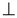AB and OQ 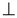CD. Join OE.

Proof: AB = CD [Given]

OP = OQ [Equal chords of a circle are equidistant from the centre]

Now in right tingles OPE and OQE,

OE = OE [Common]

Side OP = Side OQ [Proved above] 

ΔOPE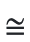ΔOQE [By RHS]

OE = QE [By cpctc]

⇒ PE –1/2AB = QE –1/2CD [ AB = CD (Given)]

⇒ PE – PB = QE – QD

⇒ EB = ED. Hence Proved.

question 11 Bisector AD of ∠BAC of ΔABC passed through the centre O of the circumcircle of ΔABC. Prove that AB = AC.

Solution: Given :Bisector AD of ∠BAC of ΔABC passed through the centre O of the circumcircle of ΔABC, 

To Prove: AB = AC.

Construction: Draw OP 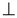AB and OQ 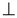AC.

Proof:

In ΔAPO and ΔAQO,

∠OPA = ∠OQA [Each = 90o (by construction)]

∠OAP = ∠OAQ [Given]

OA = OA [Common]

∴ ΔAPO≅ΔAQO [By ASS cong. prog.]

∴ OP = OQ [By cpctc]

∴ AB = AC. [ Chords equidistant from the centre are equal] Hence Proved.

THEOREM5:

The angle subtended by an arc at the centre is double the angle subtended by it at any point on the remaining part of the circle.

Given: An arc PQ of a circle subtending angles POQ at the centre O and PAQ at a point A on the remaining part of the circle.

To Prove :∠POQ = 2∠PAQ.

Construction :Join AO and extend it to a point B.

(a) (b) (c)

Proof: There arises three cases :

(a)are PQ is minor

(b)arc PQ s a semi - circle

(c)arc PQ is major.

In all the cases,

∠BOQ = ∠OAQ + ∠AQO ....(i)

[An exterior angle of triangle is equal to the sum of the two interior opposite angles]

In OAQ,

OA = OQ [Radii of a circle]

∴ ∠OAQ = ∠OQA ...(ii) [Angles opposite equal of a triangle are equal]

(i) and (ii), give,

∠BOQ = 2∠OAQ ....(iii)

Similarly,

∠BOP = 2∠OAP ....(iv)

Adding (iii) and (iv), we get

∠BOP + ∠BOQ = 2(∠OAP + ∠OAQ)

⇒ ∠POQ = 2∠PA. ....(v)

NOTE :For the case (c), where PQ is the major arc, (v) is replaced by reflex angles.

Thus, ∠POQ = 2∠PAQ.

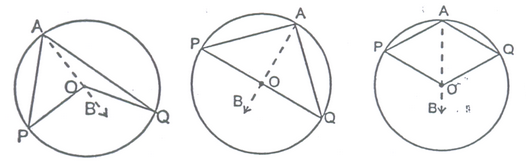
THEOREM6:

Angles in the same segment of a circle are equal.

Proof: Let P and Q be any two points on a circle to form a chord PQ, A and C any other points on the remaining part of the circle and O be the centre of the circle. Then,

∠POQ = 2∠PAQ ...(i)

And∠POQ = 2∠PCQ ...(ii) 

From above equations, we get

2∠PAQ = 2∠PCQ

⇒ ∠PAQ = ∠PCQ Hence Proved 

question 12 In the given figure, if ∠ACB = 400, ∠DPB = 1200, then y will be

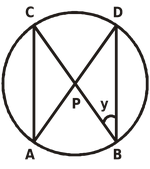
(a) 400 (b) 200 (c) 00 (d) 600

Solution: ADB = ACB = 400 (Angles in the same segment)

⇒ PDB = 400  ⇒ DPB = 1200

In ∆ DPB, PBD + DPB + PDB = 1800

PBD + 1200 + 400 = 1800

PBD + 1600 = 1800

PBD = 1800 – 1600 = 200

y = 200

THEOREM7:

Angle in the semicircle is a right angle.

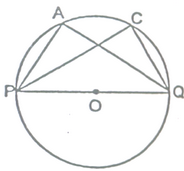
Proof :∠PAQ is an angle in the segment, which is a semicircle. 

∴ ∠PAQ = 1/2∠PAO = 1/2 × 180^o = 90^o

[∴∠PQR is straight line angle or ∠PQR = 180^o]

If we take any other point C on the semicircle, then again we get

∠PCQ = 1/2∠POQ = 1/2 × 180^o = 90^o Hence Proved.

THEOREM8:

If a line segment joining two points subtend equal angles at two other points lying on the same side of the lien containing the line segment the four points lie on a circle (i.e., they are concyclic).

Given :AB is a line segment, which subtends equal angles at two points C and D. i.e., ∠ACB = ∠ADB.

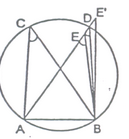
To Prove :The points A, B, C and D lie on a circle.

Proof :Let us draw a circle through the points A, C and B.

Suppose it does not pass through the point D.

Then it will intersect AD (or extended AD) at a point, say E (or E’).

If points A,C,E and B lie on a circle,

∠ACD = ∠AEB [∴ Angles in the same segment of circle are equal]

But it is given that ∠ACB = ∠ADB

Therefore, ∠AEB = ∠ADB

This is possible only when E coincides with D. [As otherwise ∠AEB >∠ADB]

Similarly, E’ should also coincide with D. So A, B, C and D are concyclic. Hence Proved.

question 13. In the given figure, PR is the diameter of the circle with centre O. ∠QPS = 400 and ∠PQT = 300. Then ∠TUR is

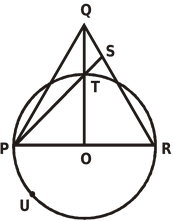
(a) 400 (b) 500 (c) 600 (d) 700

Solution: QTS = QPS + PQT = 400 + 300 = 700

( As exterior angle QTS for triangle QPT is equal to sum of interior opposite angles)

QTS = PTO = 700 (both are vertically opposite angles)

As PR is the diameter of the circle, PTR = 900, 

RTO = PTR – PTO = 900 – 700 = 200

TUR = 1/2TOR =1/2(1800 – OTR – ORT) = 1/2 (1800 – 2OTR) (As OT = OR)

= 1/2 (1800 – 400) = 700

question 14. In the given figure, CAB = 800, ABC = 400. The sum of DAB + ABD is equal to

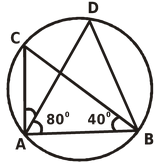
(a) 800 (b) 1000 (c) 1200 (d) 1400

Solution: Given CAB = 800, ABC = 400

from triangle ABC, ACB = 600

ADB = 600 ( Angles in the same segment are equal )

In ∆ ABD, ADB + DAB + DBA = 1800

DAB + DBA = (1800 – 600) = 1200

CYCLIC QUADRILATERAL

A quadrilateral ABCD is called cyclic if all the four vertices of it lie on a circle.