Motion In One Dimension

Displacement

The displacement of a particle is defined as the difference between its final position and its initial position. We represent the displacement as Δx.

Δx = xf− xi

The subscripts iand f refer to be initialand final positions. These are not necessarily the positions from which the particle starts its motion nor where its motion ceases. The i and f designate the particular initial and final positions we are considering out of the entire motion of the object. Notethe order : final position minus initial position . Whenever we calculate “delta” anywhere, we always take the final value minus the initial value.

Average Velocity and Average Speed

The average velocity of an object travelling along the x−axis is defined as the ratio of its displacement to the time taken for that displacement.

vav= 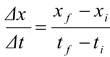(7.1)

The average speed of a particle is defined as the ratio of the total distance travelled to the time taken.

Average speed = Total distance travelled/Δt

Note that velocity and speed have different meanings.

Example : 7.1

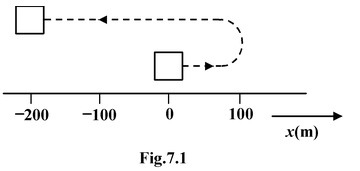
A bird flies toward east at 10 m/s for 100 m. It then turns around and flies at 20 m/s for 15 s. Find

(a) its average speed

(b) its average velocity

Δt= Δt₁+ Δt₂= 25 s

The bird flies 100 m east and then (20 m/s) (15 s) = 300 m west

(a)Average speed = Distance/Δt = 100m + 300 m/25s = 16m/s

(b)The net displacement is

Δx= Δx₁+ Δx₂= 100 m − 300 m = −200 m

So that

vav= Δx/Δt = -200m/25s = -8  m/s

The negative sign means that vavis directed toward the west.

Example: 7.2

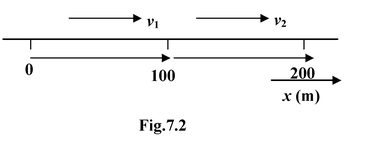
A jogger runs his first 100 m at 4 m/s and the second 100 m at 2 m/s in the same direction. What is the average velocity ?

The first half took

Δt1= (100 m)/(4 m/s) = 25 s,

while the second took

Δt1= (100 m)/(2 m/s) = 50 s,

The total time interval is

Δt= Δt₁+ Δt₂= 75 s

Therefore, his average velocity is

vav= Δx/Δt = 200m/75s = 2.67 m/s

Since 2.67 ≠ 1/2 (4+2), we see that the average velocity is not, in general, equal to the average of the velocities.

Average Acceleration is defined as the ratio of the change in velocity to the time taken.

a_av= 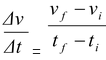(7.2)

Instantaneous Velocity is defined as the value approached by the average velocity when the time interval for measurement becomes closer and closer to zero, i.e. Δt → 0. Mathematically

v(t) =

The instantaneous velocity function is the derivative with respect to the time of the displacement function.

v(t)= 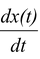(7.3)

Instantaneous Acceleration is defined analogous to the method for defining instantaneous velocity. That is, instantaneous acceleration is the value approached by the average acceleration as the time interval for the measurement becomes closer and closer to zero.

The Instantaneous acceleration function is the derivative with respect to time of the velocity function

a(t)= 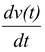(7.4)

Example: 7.3

The position of a particle is given by

x= 40 − 5t− 5t₂, where x is in metre and t is in second

(a) Find the average velocity between 1 s and 2 s

(b) Find its instantaneous velocity at 2 s

(c) Find its average acceleration between 1 s and 2 s

(d) Find its instantaneous acceleration at 2 s

Solution

(a) Att = 1 s; xi= 30 m

t = 2 s; xf= 10 m

vav= 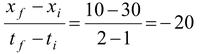m/s

(b) v = dx/dt = -5 -10t

At t= 2 s; v= −5−10(2) = −25 m/s

(c) At t = 1 s; v = −5−10 (1) = −15 m/s

t = 2 s; v = −5−10 (1) = −25 m/s

aav= 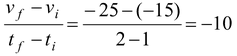m/s2

(d)a= dv/dt = −10 m/s²