Continuity of A Function
continuity and differentiability of Class 12
Definition
A function f(x) is said to be continuous at the point x = a if
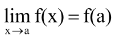
i.e., 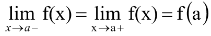 .
.
i.e., RHL = LHL = functional value.
The function is said to be continuous in a certain interval [a, b] if f(x) is continuous at every point of the interval. The continuity at boundary points is ascertained by f(b-) = f(b) & f(a) = f(a+).
- Continuity of A Function
- Discontinuity of A Function
- Derivatives
- Differentiability and Continuity
- Some Standard Differentials
- Derivative of Parametric and Logarithmic Functions
- Some Standard Substitutions
- Exercise 1
- Exercise 2
- Exercise 3
- Exercise 4
- Exercise 5
- Exercise 6
- Exercise 7
- Exercise 8
- Exercise 9
- Exercise 10









