Dipole
Electrostatics of Class 12
The arrangement of a pair of equal and opposite charges separated by some distance is called an electric dipole. Any molecule in which the centres of the positive and negative charges do not coincide may, to a first approximation, be treated as a dipole. Molecules such as HCl, CO and H2O have permanent dipoles and are called polar molecules. An electric field may also induce a charge separation in an atom or a nonpolar molecule.
Fig. (1.33 a) shows an atom as a positive point charge surrounded by a sphere of equal negative charge. When an external electric field is applied, these charges are displaced in opposite directions, as shown in Fig. (1.33 b), thereby creating an induced dipole. Such an induced dipole vanishes when the external field is removed.

|
The characteristic of a dipole is its dipole moment p. It is defined as the product of one of the charges and their separation.
It is a vector quantity that points from the negative to the positive charges. The SI unit for the electric dipole moment is Cm. |
 |
When three charges are involved as in a water molecule (Fig. 1.35) the net dipole moment is the vector sum of the two dipole moments.

Electric field intensity due to Dipole(i) Along the axis
The direction of electric field along the axis is in the same direction as that of the dipole moment. (ii) Along the bisector
|
|
The direction of electric field along the bisector is opposite to that of the dipole moment.
Electric Potential Due to a Dipole Moment
|
(i) Along the axis V|| = 2kp/x2 (1.27 a) (ii) Along the bisector V⊥ = 0 (1.27 b). |
|
|
|
Example : 1.16 Determine the electric potential and electric intensity at a distance r and at an angel θ from the axis of the dipole. Solution The dipole moment can be resolved along and perpendicular to the point A as shown. Thus V|| = 2kp cosθ/r2V⊥= 0 Hence Vnet = V|| = 2kp cosθ/r2 Similarly, |
 |
|
E|| = 2kp cosθ/r3,, E⊥ = kp sin θ/r3
The resultant electric field is
E =
Dipole in an External Uniform Field
(i) Torque
|
If a dipole is oriented at an angle θ to an uniform electric field as shown in the figure (1.39), the charges experience equal and opposite forces. So there is no net force on the dipole. However, there is a net torque on the dipole.
The magnitude of the torque is τ = pE sin θ |
 |
(ii) Potential Energy
We know that an external electric field tends to align the dipole along itself. Therefore, it takes work to rotate the dipole.
|
The work done by an external agent in rotating the dipole without change in kinetic energy is stored as potential energy. The potential energy of a dipole in an external field is given by U = − p.E (1.29) The potential energy as a function of the angle θ is shown in Fig. (1.40). The minimum potential energy occurs at θ = 0, and maximum at θ = π. The dipole is in stable equilibrium at θ = 0. If it is allowed to rotate, it oscillates about the direction of the field. |
|
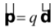
 (1.26 a)
(1.26 a) (1.26 b)
(1.26 b)

 (1.28)
(1.28)









