Introduction

Distance Formula

The distance between two points P(x1, y1) and Q(x₂, y₂), is given

by .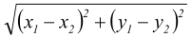

Section Formula

If R divides the join of P(x1, y₁) and Q(x₂, y₂) in the ratio m : n (m, n > 0), then

x = 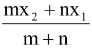y = 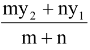(Internal Division)

and x = 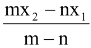y = 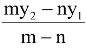(External Division)

Area of a Triangle

The area of a triangle ABC with vertices A(x₁, y₁), B(x₂, y₂) and C(x₃, y₃) denoted conventionally by the symbol Δ is given by

Δ = 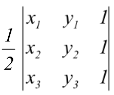= {x1(y₂ − y₃) + x²(y₃ − y₁) + x³(y₁ − y₂)}

I. If one vertex say C(x₃, y₃) is the origin (0, 0) then Δ = |x₁y₂ − x₂y1|.

II. If a₁x + b₁y + c₁ = 0, a₂x + b₂y + c₂ = 0 and a₃x + b₃y + c₃ = 0 are the sides of a triangle then the area of a triangle is given by

Δ =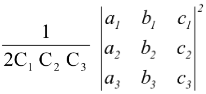

where C₁ C₂ C₃ are the cofactors of c₁ c₂ c₃ in the determinant.